Electrons are arranged in shells around an atomic nucleus. But why is this? Luckily there is is some basic mathematics that can give us a clue…
Hi everyone, in this video I wanted to discuss the basic mathematics of the Pauli Exclusion Principle. This is a fundamental principle of quantum mechanics that explains why electrons are arranged in shells (rather than all falling down into the same / lowest energy level).
To begin with, we need to consider a pair of particles that are indistinguishable. Not just identical in every way (same mass, same charge, etc), but we cannot tell them apart. If we have a system with two particles labelled A and B, some time later we should not be able to tell which is A and which is B.
If such particles exist, then the square modulus of their quantum wave function must be identical whether we find them in the orientation AB or BA. The reason for this is that the square modulus of the quantum wave function is something we can physically measure in real life. And if the value of this quantity changed depending on the orientation of the particles, then they would no longer be indistinguishable. We would be able to tell which orientation they were in.
The square modulus being equal upon “particle exchange” leads to two very simple mathematical conditions for the wave function of our system. One condition is that the wave function must not change as the particles are switched. This is known as a symmetric wave function, and applies to bosons, as discussed in my Bose-Einstein Condensate video. The other condition is that the wave function becomes negative when the particles are switched. This is known as an antisymmetric wave function, and applies to fermions (such as the electrons we will discuss).
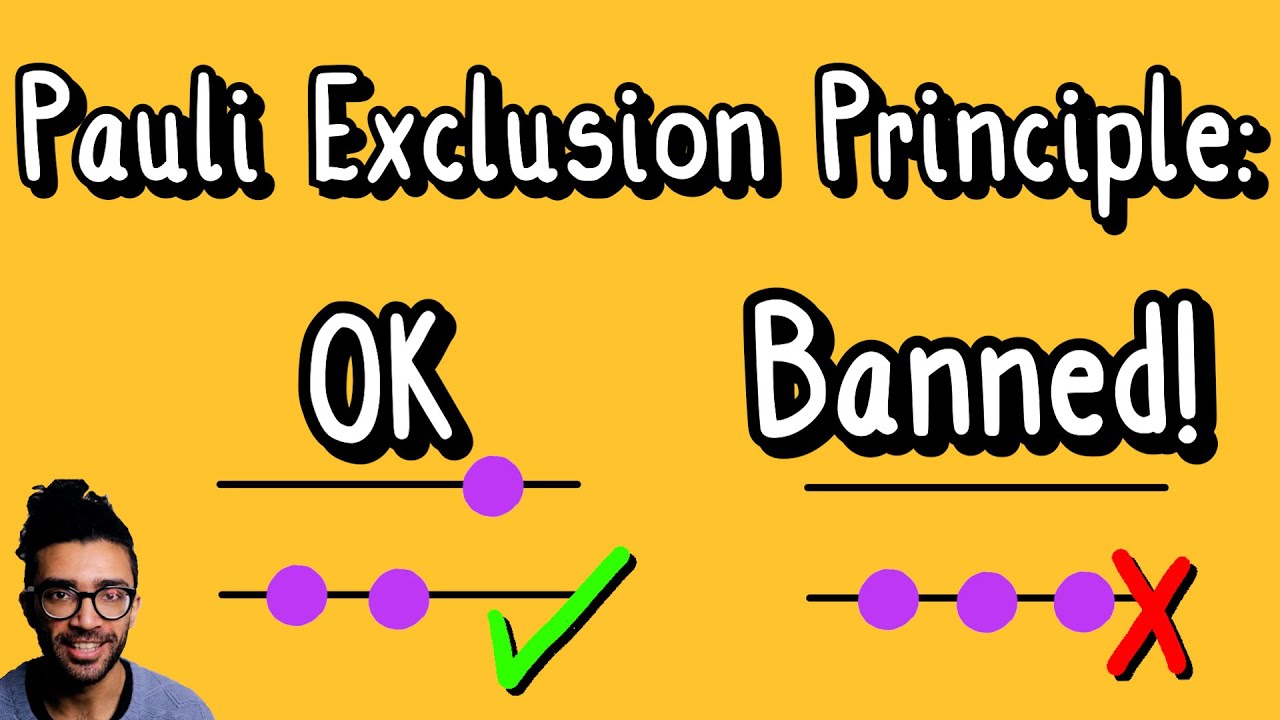
For a system containing two or more fermions, we interestingly find that no two fermions can be in the same quantum state. We visualize this in the video with a simple description of a two-level, two-electron system. And this is the Pauli Exclusion Principle!
A particle’s quantum state can be described with a series of “quantum numbers”. One of them is the principle quantum number (n), referring to which energy level the particle is in. Another is the spin quantum number (m_s), referring to whether the particle is found in the spin up or spin down state.
There are a series of numbers that are needed in order to fully describe the particle’s quantum state, and Pauli’s Exclusion Principle says that no two particles can have all the same numbers – at least one number must be different.
And this explains why electrons in atoms are arranged in shells where there is a “maximum” number of electrons that can be found in each shell – 2 for the first shell, 8 for the second shell, and so on. If electrons were bosons instead, all of them would fall to the lowest energy level. And this would not be good for the universe as we know it – chemistry would be very different, and therefore biology (and life) as we know it would probably not exist.
Bra and ket notation video:
My Quantum Mechanics playlist:
Many of you have asked about the stuff I use to make my videos, so I’m posting some affiliate links here! I make a small commission if you make a purchase through these links.
A Quantum Physics Book I Enjoy:
My camera (Canon EOS M50):
My Lens (Canon EF-M 22mm):
Microphone and Stand (Fifine):
Thanks so much for watching – please do check out my socials here:
Instagram – @parthvlogs
Patreon – patreon.com/parthg